
Armor piercing bullets do not fragment and therefore are much harder to stop. If it is a rapidly fragmenting bullet, it may be slowed down.
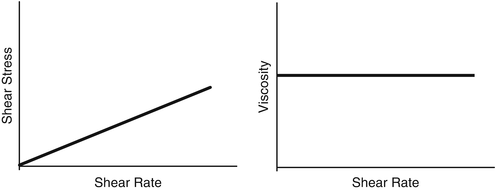
The bullet does not have much surface area when encountered straight on. The bullet is moving so fast that the soldier would need a suit of armor about five feet thick to stop a bullet. Just so you know, non-Newtonian fluids will not stop a bullet. What's the density of cornstarch and water? anon84008 May 13, 2010 Why it is called non-newtonian fluid? anon86256 May 24, 2010 Thanks, Vinoth KG anon97759 July 21, 2010 is mercury a non-newtonian fluid? if not, explain to me. taufiq, university of indonesia anon110116 yesterday So, are there any newtonian fluids in this world? if there are, can you tell me what they are? thanks. RE: Why it is called non-newtonian fluid? The fluids do not follow the laws that Newton discovered about the behavior of fluids, hence "non-newtonian." -The Answer-er RE: Any newtonian fluids in this world? There are absolutely tons of newtonian fluids. Hopefully you haven't attempted to use it in that way. RE: Able to stop a bullet? As far as Mythbusters has done, the answer is no. It is not a polymer, but ratherm an element, and for something to be a non-newtonian fluid it must be a polymer. RE: mercury a non-newtonian fluid? Mercury is a newtonian fluid. I would like to answer tons of peoples' questions. Humans have a Non-newtonian fluid that runs throughout our bodies. Why do non newtonian fluids change their viscosity under stress? anon156036 February 25, no26: Non-newtonian liquid running through our body is blood. I have to do a report on this and I'm as lost as all get out. Does anybody know what these are or where I can find information about them please? anon235065 December 15, 2011Īm I the only one completely lost? I didn't get half of this. The derivative of a field with respect to a fixed position in space is called the Eulerian derivative, while the derivative following a moving parcel is called the advective or material (or Lagrangian ) derivative.Can somebody please inform me of a point which I am struggling with? I get the Newtonian and Non-Newtonian fluids but the question which I am trying to answer on my assignment also refers to ideal solids and ideal fluids. One can measure a given property by either carrying out the measurement on a fixed point in space as particles of the fluid pass by, or by following a parcel of fluid along its streamline. The control volume can remain fixed in space or can move with the fluid.Ĭhanges in properties of a moving fluid can be measured in two different ways. This finite volume is denoted by Ω and its bounding surface ∂Ω. Sometimes it is necessary to consider a finite arbitrary volume, called a control volume, over which these principles can be applied. The equations are derived from the basic principles of continuity of mass, conservation of momentum, and conservation of energy.

Another necessary assumption is that all the fields of interest including pressure, flow velocity, density, and temperature are at least weakly differentiable. The Navier–Stokes equations are based on the assumption that the fluid, at the scale of interest, is a continuum – a continuous substance rather than discrete particles. The intent of this article is to highlight the important points of the derivation of the Navier–Stokes equations as well as its application and formulation for different families of fluids. ( January 2021) ( Learn how and when to remove this template message) Use the lead layout guide to ensure the section follows Wikipedia's norms and is inclusive of all essential details. The lead section of this article may need to be rewritten.


 0 kommentar(er)
0 kommentar(er)
